


Next: Remarks on the theoretical
Up: Inviscid Burgers
Previous: Inviscid Burgers
Contents
For the special case
![$ K(t,x)=K(x)=K\mathbbm{1}_{[a,b]}(x)$](img382.png) , where
, where 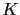 is a constant and
is a constant and ![$ [a,b]$](img383.png) is a sub-interval of
is a sub-interval of ![$ [0,1]$](img335.png) , we have
, we have
 |
(3.45) |
where
 |
(3.46) |
is the time during which the characteristic curve
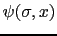 with foot
with foot 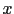 of equation (3.39-F) with
of equation (3.39-F) with  lies in the support of
lies in the support of 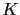 .
.
The system is then observable if and only if the function  has a non-zero lower bound, i.e.
has a non-zero lower bound, i.e.
 , the observability being defined by (see e.g. [93]):
, the observability being defined by (see e.g. [93]):
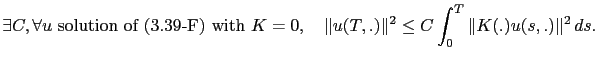 |
(3.47) |
In this case, proposition 3.2 proves the global exponential decrease of the error, provided 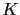 is larger than
is larger than
 , where
, where  is defined by equation (3.41).
is defined by equation (3.41).
From this remark, we can easily deduce that if for each iteration, both in the forward and backward integrations, the observability condition is satisfied, then the algorithm converges and the error decreases exponentially to 0
. Note that this is not a necessary condition, as even if  , the last exponential of equation (3.45) is bounded.
, the last exponential of equation (3.45) is bounded.



Next: Remarks on the theoretical
Up: Inviscid Burgers
Previous: Inviscid Burgers
Contents
Back to home page

![]() has a non-zero lower bound, i.e.
has a non-zero lower bound, i.e.
![]() , the observability being defined by (see e.g. [93]):
, the observability being defined by (see e.g. [93]):
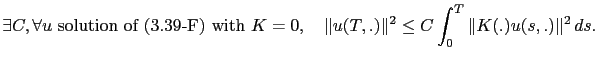
![]() , the last exponential of equation (3.45) is bounded.
, the last exponential of equation (3.45) is bounded.